sufr_random_numbers Module Reference

Procedures to generate random numbers. More...

Functions/Subroutines
integer function	get_ran_seed (degree, date_array)
	Use the system clock to get a random initialisation seed (i.e., negative integer) for a random-numbed generator.
real(double) function	ran_unif (seed)
	Generate a pseudo-random number from a uniform distribution 0 < r < 1.
real(double) function	ran_gauss (seed)
	Generate a pseudo-random number from a Gaussian distribution with mean 0 and standard deviation 1.
subroutine	ran_str (seed, str)
	Generate a pseudo-random number from a uniform distribution 0 < r < 1.

Detailed Description

Procedures to generate random numbers.

Function/Subroutine Documentation

get_ran_seed()

integer function sufr_random_numbers::get_ran_seed	(	integer, intent(in)	degree,
		integer, dimension(8), intent(in), optional	date_array )

Use the system clock to get a random initialisation seed (i.e., negative integer) for a random-numbed generator.

Parameters

degree	Degree of randomness: 0-completely (same result for a ms), 1-same result during a clock hour, 2-same result during a calendar day (int)
date_array	Provide a date/time array as provided by date_and_time() to generate a non-random seed, e.g. for reproduction purposes ([year,month,day, (tz), hour,minute,second,millisecond]; optional)

Return values

get_ran_seed

Randon-number seed: -1e6 < seed < 0 (int)

Definition at line 40 of file random_numbers.f90.

References get_ran_seed().

Referenced by sufr_system::execute_command_line_and_return_str(), and get_ran_seed().

Here is the call graph for this function:

ran_gauss()

real(double) function sufr_random_numbers::ran_gauss

(

integer, intent(inout)

seed

)

Generate a pseudo-random number from a Gaussian distribution with mean 0 and standard deviation 1.

Parameters

seed	The seed to generate the random number from

Return values

ran_gauss

The random number, using a Gaussian distribution

• Two pseudo-random numbers are drawn from a uniform distribution, using ran_unif().
• These are converted into two pseudo-random numbers from a Gaussian distribution using the Box-Muller transform
• Odd-numbered calls return the first, even-numbered calls the second number
  • using the polar form is ~38% faster than using the basic form

See also

e.g.:

• Numerical Recipes in Fortran 77, Sect.7.2.
• http://en.wikipedia.org/wiki/Box%E2%80%93Muller_transform

Definition at line 148 of file random_numbers.f90.

References sufr_kinds::double, ran_gauss(), and ran_unif().

Referenced by ran_gauss().

Here is the call graph for this function:

ran_str()

subroutine sufr_random_numbers::ran_str	(	integer, intent(inout)	seed,
		character, dimension(*), intent(inout)	str )

Generate a pseudo-random number from a uniform distribution 0 < r < 1.

Parameters

seed	The seed to generate the random number from. Set seed<0 to initialise the generator; seed is updated between calls (int).
str	The random string (I/O: output str has length of input str, filled with random characters)

Definition at line 188 of file random_numbers.f90.

References ran_unif().

Here is the call graph for this function:

ran_unif()

real(double) function sufr_random_numbers::ran_unif

(

integer, intent(inout)

seed

)

Generate a pseudo-random number from a uniform distribution 0 < r < 1.

Parameters

seed	The seed to generate the random number from. Set seed<0 to initialise the generator; seed is updated between calls (int).

Return values

ran_unif

The random number, uniformely generated between 0 < r < 1 (double).

• Use two L'Ecuyer generators, period is
• tab is a Bays-Durham shuffle table of length Ntab

  See also

  Numerical Recipes in Fortran 77, Sect.7.1.

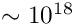
Definition at line 80 of file random_numbers.f90.

References sufr_kinds::dbl, sufr_kinds::double, and ran_unif().

Referenced by sufr_system::execute_command_line_and_return_str(), ran_gauss(), ran_str(), and ran_unif().

Here is the call graph for this function: